

Fractal Dimension Index o índice de dimensión fractal. El Fractal Dimension Index (o índice de dimensión fractal) se basa en la idea de que los mercados financieros son parte de un proceso natural y no mecánico, y por tanto son caóticos.

Es por eso por lo que deben usarse herramientas no lineales para predecir la dinámica de los mercados, y aquí es donde entra la geometría fractal. Todos los sistemas caóticos tienen una medida cuantitativa que se conoce como “dimensión fractal”. La dimensión fractal describe cómo el objeto ocupa su lugar en el espacio. Un fractal es un objeto en el que sus partes individuales son similares al conjunto. Por eso, los fractales mantienen sus dimensiones independientemente de la escala que se utilice. What the History of Math Can Teach Us About the Future of AI. Mathematicians Measure Infinities, and Find They're Equal. El genio babilonio que se adelantó a Pitágoras 1.000 años y creó las tablas de trigonometría más precisas del mundo - BBC Mundo.
The Tao of Tau - Scientific American Blog Network. “It is lamentable that there’s no famous dessert named ‘tau,’” Michael Hartl told me recently at a sunny, stylish café in Venice, California.
He reluctantly admitted that pi, the constant approximately equal to 3.14, has this one advantage over tau, a number he introduced to replace it. Pastry puns aside, Hartl has achieved minor internet fame for arguing that tau is superior to its vastly better known cousin. In his popular 2010 “Tau Manifesto,” inspired by Bob Palais’ 2001 essay “Pi Is Wrong,” Hartl posits that pi, the ratio of a circle’s circumference to its diameter, creates unnecessary complications in many formulas. A more appropriate number to work with when it comes to circles would be 2pi, or about 6.28. The Most Mathematically Perfect Day of the Year - Scientific American Blog Network. Whether you write it 6/28 or 28/6, today is a perfect day.
A perfect number is a number that is the sum of its factors besides itself, and 6 (1+2+3) and 28 (1+2+4+7+14) are the first two perfect numbers. Hence, June 28 is a perfect day. Perfect numbers are few and far between, so don't hold out for a celebration in which the year is perfect as well. The last time that happened was in 496, and the next time is over 6,000 years away, in 8128. Mathematical date enthusiasts will note that today is also Tau Day, if you didn’t get enough circle constant celebration in March. If you’d prefer to keep the celebration mathematical, you could of course go looking for the next perfect number. En el Día de Pi, 10 curiosidades sobre el número irracional. El matemático griego Arquímedes fue uno de los primeros en aproximar su valor.
Para aquellos que deseen conocer cuánto mide, solo deberán calcular el perímetro de una circunferencia y dividirlo por su diámetro. Discovery of classic pi formula a ‘cunning piece of magic’ : NewsCenter. While most people associate the mathematical constant π (pi) with arcs and circles, mathematicians are accustomed to seeing it in a variety of fields.

But two University scientists were still surprised to find it lurking in a quantum mechanics formula for the energy states of the hydrogen atom. “We didn’t just find pi,” said Tamar Friedmann, a visiting assistant professor of mathematics and a research associate of high energy physics, and co-author of a paper published this week in the Journal of Mathematical Physics. “We found the classic seventeenth century Wallis formula for pi, making us the first to derive it from physics, in general, and quantum mechanics, in particular.” The Wallis formula—developed by British mathematician John Wallis in his book Arithmetica Infinitorum—defines π as the product of an infinite string of ratios made up of integers.
Friedmann did not set out to look for π nor for the Wallis formula. Which can be reduced to the classic Wallis formula. Einstein notation - Wikipedia. Pi Day · Celebrate Mathematics on March 14th. Levi-Civita symbol. Ricci calculus. Mathematical Beauty: A Q&A with Fields Medalist Michael Atiyah. Despite Michael Atiyah’s many accolades — he is a winner of both the Fields and the Abel prizes for mathematics; a past president of the Royal Society of London, the oldest scientific society in the world (and a past president of the Royal Society of Edinburgh); a former master of Trinity College, Cambridge; a knight and a member of the royal Order of Merit; and essentially Britain’s mathematical pope — he is nonetheless perhaps most aptly described as a matchmaker.
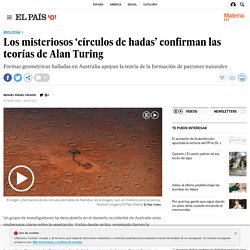
He has an intuition for arranging just the right intellectual liaisons, oftentimes involving himself and his own ideas, and over the course of his half-century-plus career he has bridged the gap between apparently disparate ideas within the field of mathematics, and between mathematics and physics. Penrose had been trying to develop his “twistor” theory, a path toward quantum gravity that’s been in the works for nearly 50 years. “I thought, ‘Oh, my God,’” Penrose said. Los misteriosos ‘círculos de hadas’ confirman las teorías de Alan Turing. Un grupo de investigadores ha descubierto en el desierto occidental de Australia unos misteriosos claros entre la vegetación.
Math Mystery: Shinichi Mochizuki and the Impenetrable Proof. Sometime on the morning of August 30 2012, Shinichi Mochizuki quietly posted four papers on his website.

The papers were huge—more than 500 pages in all—packed densely with symbols, and the culmination of more than a decade of solitary work. They also had the potential to be an academic bombshell. The Golden Ratio: Design's Biggest Myth. In the world of art, architecture, and design, the golden ratio has earned a tremendous reputation.
Greats like Le Corbusier and Salvador Dalí have used the number in their work. The Parthenon, the Pyramids at Giza, the paintings of Michelangelo, the Mona Lisa, even the Apple logo are all said to incorporate it. It's bullshit.