

David Richeson. The Map is Not the Territory. New Math Game: Factor Dominoes! Lately I've been looking for different ways for my seven year old and I to conceptualize multiplication.
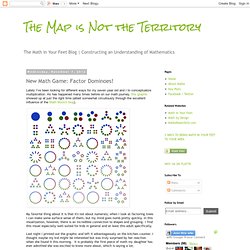
As has happened many times before on our math journey, this graphic showed up at just the right time (albeit somewhat circuitously through the excellent influence of the Math Munch blog). My favorite thing about it is that it's not about numerals; when I look at factoring trees I can make some surface sense of them, but my mind goes numb pretty quickly.
In this visualization, however, there is an incredible connection to shapes and grouping. I find this visual especially well-suited for kids in general and at least this adult specifically. Last night I printed out the graphic and left it advantageously on the kitchen counter. She wondered what it was about so we looked it over together. All Topics. Realisation of PentiDisc. Links: PentiDisc short description PentiDisc projectbeschrijving PentiDisc Intégré PentiDisc general description Cubic Fuzzy Precision short paper.

Mathematics6.jpg (1920×1200) MathPuzzle.com. Pictures of Math.
Fractals. Geometry. Science Mysteries, Fibonacci Numbers and Golden section in Nature. Yupana - an Incan Fibonacci Calculator. The term yupana, deriving from the word Quechua yupay (count),[1] is commonly defined as an abacus used to perform arithmetic operations dating back to the time of the Incas Types of yupana[edit] The term yupana refers to two distinct classes of objects: table-yupana (or archaeological yupana): a system of trays of different sizes and materials, carved at the top into geometric boxes were is assumed seeds or pebbles were be placed.
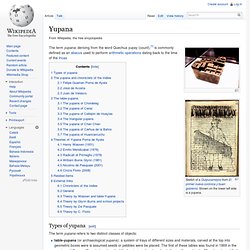
The first of these tables was found in 1869 in the province of Cuenca (Ecuador) and marked the beginning of systematic studies on these objects. All archaeological finds are very different from each other.[2]yupana of Poma de Ayala: a picture on page 360 of El primer nueva corónica y buen gobierno written by the chronicler of the Indies Poma de Ayala, representing a 5x4 chessboard.[3] The yupana and chroniclers of the Indies[edit] Several chroniclers of the Indies described, unfortunately approximately, the Incan abacus and its operation. ...
José de Acosta[edit] Node (physics) A standing wave.

The red dots are the wave nodes A node is a point along a standing wave where the wave has minimum amplitude. For instance, in a vibrating guitar string, the ends of the string are nodes. By changing the position of the end node through frets, the guitarist changes the effective length of the vibrating string and thereby the note played. Van der Pol oscillator. Evolution of the limit cycle in the phase plane.
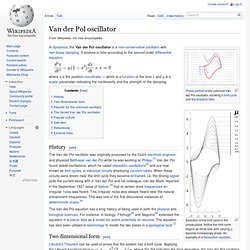
Notice the limit cycle begins as circle and, with varying μ, become increasingly sharp. An example of a Relaxation oscillator. In dynamics, the Van der Pol oscillator is a non-conservative oscillator with non-linear damping. It evolves in time according to the second order differential equation: History[edit] Two dimensional form[edit] 9 Mental Math Tricks. Math can be terrifying for many people.

Uncoiling the spiral: Maths and hallucinations. December 2009 You can read a more accessible version of this article here.
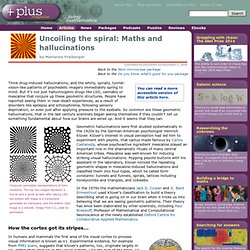
Think drug-induced hallucinations, and the whirly, spirally, tunnel-vision-like patterns of psychedelic imagery immediately spring to mind. But it's not just hallucinogenic drugs like LSD, cannabis or mescaline that conjure up these geometric structures. People have reported seeing them in near-death experiences, as a result of disorders like epilepsy and schizophrenia, following sensory deprivation, or even just after applying pressure to the eyeballs. So common are these geometric hallucinations, that in the last century scientists began asking themselves if they couldn't tell us something fundamental about how our brains are wired up.
Computer generated representations of form constants. Sin & Cos: The Programmer's Pals! Octave. GNU Octave is a high-level interpreted language, primarily intended for numerical computations.

It provides capabilities for the numerical solution of linear and nonlinear problems, and for performing other numerical experiments. It also provides extensive graphics capabilities for data visualization and manipulation. Octave is normally used through its interactive command line interface, but it can also be used to write non-interactive programs. The Octave language is quite similar to Matlab so that most programs are easily portable. Fast and usable calculator. Impress your friends with mental Math tricks » Fun Math Blog. See Math tricks on video at the Wild About Math!

Mathcasts page. Being able to perform arithmetic quickly and mentally can greatly boost your self-esteem, especially if you don't consider yourself to be very good at Math. And, getting comfortable with arithmetic might just motivate you to dive deeper into other things mathematical. This article presents nine ideas that will hopefully get you to look at arithmetic as a game, one in which you can see patterns among numbers and pick then apply the right trick to quickly doing the calculation. LiveMath™ Software Products. An Intuitive Explanation of Fourier Theory. Steven Lehar slehar@cns.bu.edu Fourier theory is pretty complicated mathematically. But there are some beautifully simple holistic concepts behind Fourier theory which are relatively easy to explain intuitively. Step-by-Step Math Problem Solver. MathPages.