

Dirac equation. In particle physics, the Dirac equation is a relativistic wave equation derived by British physicist Paul Dirac in 1928.
In its free form, or including electromagnetic interactions, it describes all spin-½ massive particles, for which parity is a symmetry, such as electrons and quarks, and is consistent with both the principles of quantum mechanics and the theory of special relativity,[1] and was the first theory to account fully for special relativity in the context of quantum mechanics.
Although Dirac did not at first fully appreciate the importance of his results, the entailed explanation of spin as a consequence of the union of quantum mechanics and relativity—and the eventual discovery of the positron—represent one of the great triumphs of theoretical physics. Mathematical formulation[edit] The Dirac equation in the form originally proposed by Dirac is:[3] where ψ = ψ(x, t) is the wave function for the electron of rest mass m with spacetime coordinates x, t. Dirac's coup[edit] with. Klein–Gordon equation. The Klein–Gordon equation (Klein–Fock–Gordon equation or sometimes Klein–Gordon–Fock equation) is a relativistic version of the Schrödinger equation.
It is the equation of motion of a quantum scalar or pseudoscalar field, a field whose quanta are spinless particles. It cannot be straightforwardly interpreted as a Schrödinger equation for a quantum state, because it is second order in time and because it does not admit a positive definite conserved probability density. Still, with the appropriate interpretation, it does describe the quantum amplitude for finding a point particle in various places, the relativistic wavefunction, but the particle propagates both forwards and backwards in time. Any solution to the Dirac equation is automatically a solution to the Klein–Gordon equation, but the converse is not true. Statement[edit] The Klein–Gordon equation is This is often abbreviated as where and is the d'Alembert operator, defined by The equation is most often written in natural units: is written. Pauli equation. Equation[edit] For a particle of mass m and charge q, in an electromagnetic field described by the vector potential A = (Ax, Ay, Az) and scalar electric potential ϕ, the Pauli equation reads: where σ = (σx, σy, σz) are the Pauli matrices collected into a vector for convenience, p = −iħ∇ is the momentum operator wherein ∇ denotes the gradient operator, and is the two-component spinor wavefunction, a column vector written in Dirac notation. is a 2 × 2 matrix operator, because of the Pauli matrices.
Substitution into the Schrödinger equation gives the Pauli equation. The Pauli matrices can be removed from the kinetic energy term, using the Pauli vector identity: to obtain[2] where B = ∇ × A is the magnetic field. Schrödinger equation. In quantum mechanics, the Schrödinger equation is a partial differential equation that describes how the quantum state of some physical system changes with time.
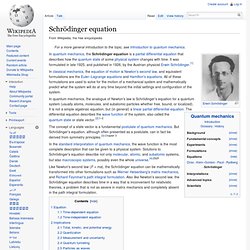
It was formulated in late 1925, and published in 1926, by the Austrian physicist Erwin Schrödinger.[1] In classical mechanics, the equation of motion is Newton's second law, and equivalent formulations are the Euler–Lagrange equations and Hamilton's equations. All of these formulations are used to solve for the motion of a mechanical system and mathematically predict what the system will do at any time beyond the initial settings and configuration of the system. In quantum mechanics, the analogue of Newton's law is Schrödinger's equation for a quantum system (usually atoms, molecules, and subatomic particles whether free, bound, or localized).
It is not a simple algebraic equation, but (in general) a linear partial differential equation. The concept of a state vector is a fundamental postulate of quantum mechanics.