

Hilbert space. The state of a vibrating string can be modeled as a point in a Hilbert space.
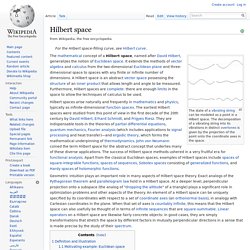
The decomposition of a vibrating string into its vibrations in distinct overtones is given by the projection of the point onto the coordinate axes in the space. Hilbert spaces arise naturally and frequently in mathematics and physics, typically as infinite-dimensional function spaces. The earliest Hilbert spaces were studied from this point of view in the first decade of the 20th century by David Hilbert, Erhard Schmidt, and Frigyes Riesz. They are indispensable tools in the theories of partial differential equations, quantum mechanics, Fourier analysis (which includes applications to signal processing and heat transfer)—and ergodic theory, which forms the mathematical underpinning of thermodynamics. John von Neumann coined the term Hilbert space for the abstract concept that underlies many of these diverse applications. Definition and illustration[edit] Motivating example: Euclidean space[edit]
Quantum chaos. Quantum chaos is a branch of physics which studies how chaotic classical dynamical systems can be described in terms of quantum theory.
The primary question that quantum chaos seeks to answer is: "What is the relationship between quantum mechanics and classical chaos? " The correspondence principle states that classical mechanics is the classical limit of quantum mechanics. If this is true, then there must be quantum mechanisms underlying classical chaos; although this may not be a fruitful way of examining classical chaos. If quantum mechanics does not demonstrate an exponential sensitivity to initial conditions, how can exponential sensitivity to initial conditions arise in classical chaos, which must be the correspondence principle limit of quantum mechanics? [1][2] In seeking to address the basic question of quantum chaos, several approaches have been employed: Stochastic interpretation. The stochastic interpretation is an interpretation of quantum mechanics.
The modern application of stochastics to quantum mechanics involves the assumption of spacetime stochasticity, the idea that the small-scale structure of spacetime is undergoing both metric and topological fluctuations (John Archibald Wheeler's "quantum foam"), and that the averaged result of these fluctuations recreates a more conventional-looking metric at larger scales that can be described using classical physics, along with an element of nonlocality that can be described using quantum mechanics. A stochastic interpretation of quantum mechanics due to persistent vacuum fluctuations is suggested by Roumen Tsekov.
The main idea is that vacuum (or spacetime) fluctuations are the reason for quantum mechanics and not a result of it how it is usually considered. See also[edit] References[edit] Edward Nelson (1966). Density matrix. Explicitly, suppose a quantum system may be found in state with probability p1, or it may be found in state with probability p2, or it may be found in state with probability p3, and so on.
The density operator for this system is[1] By choosing a basis (which need not be orthogonal), one may resolve the density operator into the density matrix, whose elements are[1] For an operator (which describes an observable is given by[1] In words, the expectation value of A for the mixed state is the sum of the expectation values of A for each of the pure states Mixed states arise in situations where the experimenter does not know which particular states are being manipulated. Pure and mixed states[edit] Quantum statistical mechanics. Expectation[edit] From classical probability theory, we know that the expectation of a random variable X is completely determined by its distribution DX by assuming, of course, that the random variable is integrable or that the random variable is non-negative.
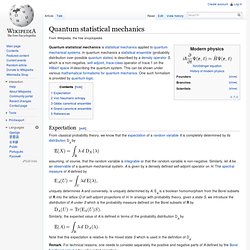
Similarly, let A be an observable of a quantum mechanical system. A is given by a densely defined self-adjoint operator on H. The spectral measure of A defined by uniquely determines A and conversely, is uniquely determined by A. Similarly, the expected value of A is defined in terms of the probability distribution DA by Note that this expectation is relative to the mixed state S which is used in the definition of DA.
Remark. One can easily show: Note that if S is a pure state corresponding to the vector ψ, then: Von Neumann entropy[edit] Of particular significance for describing randomness of a state is the von Neumann entropy of S formally defined by Actually, the operator S log2 S is not necessarily trace-class. And we define Remark.