Non-Euclidean geometry

Non-Euclidean geometry Version for printing In about 300 BC Euclid wrote The Elements, a book which was to become one of the most famous books ever written. Euclid stated five postulates on which he based all his theorems: To draw a straight line from any point to any other. Proclus (410-485) wrote a commentary on The Elements where he comments on attempted proofs to deduce the fifth postulate from the other four, in particular he notes that Ptolemy had produced a false 'proof'. Playfair's Axiom:- Given a line and a point not on the line, it is possible to draw exactly one line through the given point parallel to the line. Although known from the time of Proclus, this became known as Playfair's Axiom after John Playfair wrote a famous commentary on Euclid in 1795 in which he proposed replacing Euclid's fifth postulate by this axiom. Many attempts were made to prove the fifth postulate from the other four, many of them being accepted as proofs for long periods of time until the mistake was found.

Hyperbolic geometry Lines through a given point P and asymptotic to line R. A triangle immersed in a saddle-shape plane (a hyperbolic paraboloid), as well as two diverging ultraparallel lines. In mathematics, hyperbolic geometry (also called Lobachevskian geometry or Bolyai–Lobachevskian geometry) is a non-Euclidean geometry, meaning that the parallel postulate of Euclidean geometry is replaced. The parallel postulate in Euclidean geometry is equivalent to the statement that, in two-dimensional space, for any given line R and point P not on R, there is exactly one line through P that does not intersect R; i.e., that is parallel to R. Because there is no precise hyperbolic analogue to Euclidean parallel lines, the hyperbolic use of parallel and related terms varies among writers. A characteristic property of hyperbolic geometry is that the angles of a triangle add to less than a straight angle, or 180°. Non-intersecting lines[edit] between parallel lines yields an angle of parallelism equal to 90°. . . using

R'lyeh The location of R'Lyeh given by Lovecraft was 47°9′S 126°43′W in the southern Pacific Ocean. August Derleth placed it at 49°51′S 128°34′W. Both locations are close to the Pacific pole of inaccessibility or "Nemo" point, 48°52.6′S 123°23.6′W, a point in the ocean farthest from any land mass. The nightmare corpse-city of R'lyeh…was built in measureless eons behind history by the vast, loathsome shapes that seeped down from the dark stars. There lay great Cthulhu and his hordes, hidden in green slimy vaults. R'lyeh is characterized by bizarre architecture likened to non-Euclidean geometry. Lovecraft claims R'lyeh is located at WikiMiniAtlas 47°9′S 126°43′W / 47.150°S 126.717°W / -47.150; -126.717 (R'lyeh fictional location (Lovecraft))Coordinates: 48°52.6′S 123°23.6′W / 48.8767°S 123.3933°W / -48.8767; -123.3933 (Oceanic Pole of Inaccessibility)), a point in the ocean farthest from any land mass. See also[edit] Bloop Notes[edit] ^ Jump up to: a b c H. References[edit] External links[edit]

Cruising the Mississippi River The USACE (Army Corp of Engineers) has divided the Mississippi River into two halves. The Upper Mississippi is one half, and the Lower Mississippi is the other. Cairo is the dividing point. The Mississippi River's mouth is located 95 miles south of New Orleans at the Gulf of Mexico. Cairo, Illinois, is at Mile Maker 954 on the Lower Mississippi. For Great Loopers, the Mississippi starts at Grafton, IL where the Illinois River joins the Upper Mississippi at Mile 219.

Finsler manifold In mathematics, particularly differential geometry, a Finsler manifold is a differentiable manifold M where a (possibly asymmetric) Minkowski norm F(x, −) is provided on each tangent space TxM, that enables one to define the length of any smooth curve γ : [a, b] → M as Finsler manifolds are more general than Riemannian manifolds since the tangent norms need not be induced by inner products. Every Finsler manifold becomes an intrinsic quasimetric space when the distance between two points is defined as the infimum length of the curves that join them. Élie Cartan (1933) named Finsler manifolds after Paul Finsler, who studied this geometry in his dissertation (Finsler 1918). Definition[edit] A Finsler manifold is a differentiable manifold M together with a Finsler metric, which is a continuous nonnegative function F: TM → [0, +∞) defined on the tangent bundle so that for each point x of M, In other words, F(x, −) is an asymmetric norm on each tangent space TxM. Examples[edit] Let where Notes[edit]

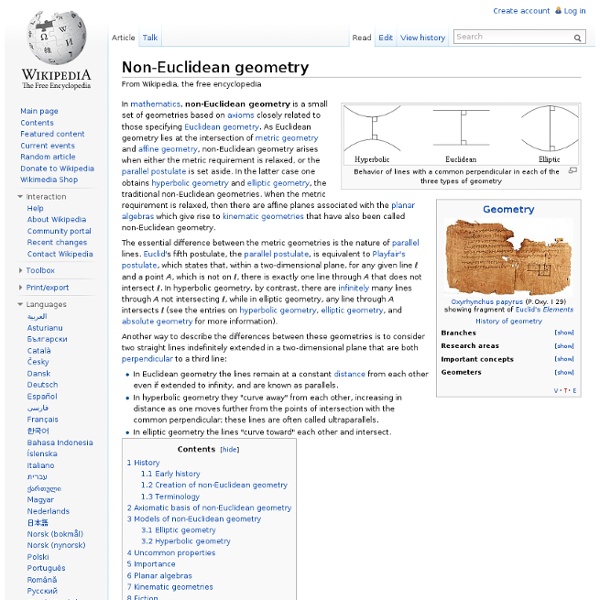
Non-Euclidean Geometry In three dimensions, there are three classes of constant curvature geometries. All are based on the first four of Euclid's postulates, but each uses its own version of the parallel postulate. The "flat" geometry of everyday intuition is called Euclidean geometry (or parabolic geometry), and the non-Euclidean geometries are called hyperbolic geometry (or Lobachevsky-Bolyai-Gauss geometry) and elliptic geometry (or Riemannian geometry). Spherical geometry is a non-Euclidean two-dimensional geometry. It was not until 1868 that Beltrami proved that non-Euclidean geometries were as logically consistent as Euclidean geometry. Elliptic geometry Definitions[edit] In elliptic geometry, two lines perpendicular to a given line must intersect. In fact, the perpendiculars on one side all intersect at the absolute pole of the given line. The perpendiculars on the other side also intersect at a point, which is different from the other absolute pole only in spherical geometry, for in elliptic geometry the poles on either side are the same. There are no antipodal points in elliptic geometry. The distance between a pair of points is proportional to the angle between their absolute polars.[2] As explained by H. Two dimensions[edit] The spherical model[edit] On a sphere, the sum of the angles of a triangle is not equal to 180°. A simple way to picture elliptic geometry is to look at a globe. More precisely, the surface of a sphere is a model of elliptic geometry if lines are modeled by great circles, and points at each other's antipodes are considered to be the same point. Comparison with Euclidean geometry[edit] . Elliptic space[edit] or

Hastur Hastur (The Unspeakable One, Him Who Is Not to be Named, Assatur, Xastur, H'aaztre, or Kaiwan) is an entity of the Cthulhu Mythos. Hastur first appeared in Ambrose Bierce's short story "Haïta the Shepherd" (1893) as a benign god of shepherds. Hastur is briefly mentioned in H.P. Lovecraft's The Whisperer in Darkness; previously, Robert W. In Terry Pratchett and Neil Gaiman's book Good Omens Hastur appears as a fallen angel and duke of hell. Hastur in the mythos[edit] In Bierce's "Haïta the Shepherd", which appeared in the collection Can Such Things Be? H. It is unclear from this quote if Lovecraft's Hastur is a person, a place, an object (such as the Yellow Sign), or a deity (this ambiguity is recurrent in Lovecraft's descriptions of the mythic entities). In "Supernatural Horror In Literature" (written 1926–27, revised 1933, published in The Recluse in 1927), when telling about "The Yellow Sign" by Chambers, H. In Chambers' "The Yellow Sign" the only mentioning of Hastur is: See also[edit]

I, Libertine I, Libertine was a literary hoax novel that began as a practical joke by late-night radio raconteur Jean Shepherd. Creation of the hoax[edit] Shepherd was highly annoyed at the way that the bestseller lists were being compiled in the mid-1950s. Publication[edit] Bookstores became interested in carrying Ewing's novel, which allegedly had been banned in Boston. A few weeks before publication, The Wall Street Journal exposed the hoax, already an open secret.[2] Plot[edit] Rife with jokes and wordplay, the novel can still be read as an entertaining historical romance. Cover painting[edit] The front cover displays a quote: "'Gadzooks,' quoth I, 'but here's a saucy bawd!'". See also[edit] J. References[edit] External links[edit]

Riemannian geometry Riemannian geometry is the branch of differential geometry that studies Riemannian manifolds, smooth manifolds with a Riemannian metric, i.e. with an inner product on the tangent space at each point that varies smoothly from point to point. This gives, in particular, local notions of angle, length of curves, surface area and volume. From those, some other global quantities can be derived by integrating local contributions. Riemannian geometry originated with the vision of Bernhard Riemann expressed in his inaugural lecture "Ueber die Hypothesen, welche der Geometrie zu Grunde liegen" ("On the Hypotheses on which Geometry is Based"). Introduction[edit] Every smooth manifold admits a Riemannian metric, which often helps to solve problems of differential topology. There exists a close analogy of differential geometry with the mathematical structure of defects in regular crystals. The following articles provide some useful introductory material: Classical theorems[edit] General theorems[edit]

Body updated version is published in Mathematical Intelligencer, Vol. 23, No. 2, pp. 17-28, Spring 2001. David W. Henderson Department of Mathematics, Cornell University, Ithaca, NY, USA, dwh2@cornell.edu Daina Taimiða Department of Mathematics, Cornell University, dtaimina@math.cornell.edu For God's sake, please give it up.  Wolfgang Bolyai urging his son János Bolyai to give up work on hyperbolic geometry. In June of 1997, Daina was in a workshop watching the leader of the workshop, David, helping the participants study ideas of hyperbolic geometry using a paper and tape surface in much the same way that one can study ideas of spherical geometry by using the surface of a physical ball. But, Wait! Constructions of Hyperbolic Planes We will describe three different isometric constructions of the hyperbolic plane (or approximations to the hyperbolic plane) as surfaces in 3-space. 1. This is the paper and tape surface that David learned from William Thruston. Figure 1. 2. Figure 2. Figure 3. 3. 1.

Taxicab geometry Taxicab geometry versus Euclidean distance: In taxicab geometry all three pictured lines (red, yellow, and blue) have the same length (12) for the same route. In Euclidean geometry, the green line has length , and is the unique shortest path. Taxicab geometry, considered by Hermann Minkowski in 19th century Germany, is a form of geometry in which the usual distance function or metric of Euclidean geometry is replaced by a new metric in which the distance between two points is the sum of the absolute differences of their Cartesian coordinates. norm (see Lp space), city block distance, Manhattan distance, or Manhattan length, with corresponding variations in the name of the geometry.[1] The latter names allude to the grid layout of most streets on the island of Manhattan, which causes the shortest path a car could take between two intersections in the borough to have length equal to the intersections' distance in taxicab geometry. Formal definition[edit] The taxicab distance, where are vectors

Cthulhu Cthulhu[1] is a fictional cosmic entity that first appeared in the short story "The Call of Cthulhu", published in the pulp magazine Weird Tales in 1928. The character was created by writer H. P. Lovecraft. Spelling and pronunciation[edit] Appearance[edit] In "The Call of Cthulhu", H. Publication history[edit] H. August Derleth, a correspondent of Lovecraft, used the creature's name to identify the system of lore employed by Lovecraft and his literary successors: the Cthulhu Mythos. According to Derleth's scheme, "Great Cthulhu is one of the Water Beings" and was engaged in an age-old arch-rivalry with a designated Air elemental, Hastur the Unspeakable, described as Cthulhu's "half-brother".[13] Based on this framework, Derleth wrote a series of short stories published in Weird Tales 1944–1952 and collected as The Trail of Cthulhu, depicting the struggle of a Dr. Derleth's interpretations have been criticized by Lovecraft enthusiast Michel Houellebecq. Legacy[edit] See also[edit]