

Continuum hypothesis. This article is about the hypothesis in set theory.

For the assumption in fluid mechanics, see Fluid mechanics. In mathematics, the continuum hypothesis is a hypothesis about the possible sizes of infinite sets. It states: There is no set whose cardinality is strictly between that of the integers and the real numbers. Cardinality of infinite sets[edit] Two sets are said to have the same cardinality or cardinal number if there exists a bijection (a one-to-one correspondence) between them.
Has the same cardinality as With infinite sets such as the set of integers or rational numbers, this becomes more complicated to demonstrate. Cantor gave two proofs that the cardinality of the set of integers is strictly smaller than that of the set of real numbers (see Cantor's first uncountability proof and Cantor's diagonal argument). The hypothesis states that the set of real numbers has minimal possible cardinality which is greater than the cardinality of the set of integers. For which greater than . . Gödel's incompleteness theorems. Gödel's incompleteness theorems are two theorems of mathematical logic that establish inherent limitations of all but the most trivial axiomatic systems capable of doing arithmetic.
The theorems, proven by Kurt Gödel in 1931, are important both in mathematical logic and in the philosophy of mathematics. The two results are widely, but not universally, interpreted as showing that Hilbert's program to find a complete and consistent set of axioms for all mathematics is impossible, giving a negative answer to Hilbert's second problem.
The first incompleteness theorem states that no consistent system of axioms whose theorems can be listed by an "effective procedure" (i.e., any sort of algorithm) is capable of proving all truths about the relations of the natural numbers (arithmetic). For any such system, there will always be statements about the natural numbers that are true, but that are unprovable within the system. Background[edit] First incompleteness theorem[edit] Diagonalization[edit] B. Ranking. By reducing detailed measures to a sequence of ordinal numbers, rankings make it possible to evaluate complex information according to certain criteria.
Thus, for example, an Internet search engine may rank the pages it finds according to an estimation of their relevance, making it possible for the user quickly to select the pages they are likely to want to see. Analysis of data obtained by ranking commonly requires non-parametric statistics. Strategies for assigning rankings[edit] It is not always possible to assign rankings uniquely. For example, in a race or competition two (or more) entrants might tie for a place in the ranking.
A common shorthand way to distinguish these ranking strategies is by the ranking numbers that would be produced for four items, with the first item ranked ahead of the second and third (which compare equal) which are both ranked ahead of the fourth. Standard competition ranking ("1224" ranking)[edit] Modified competition ranking ("1334" ranking)[edit] Matrix (mathematics) Each element of a matrix is often denoted by a variable with two subscripts.
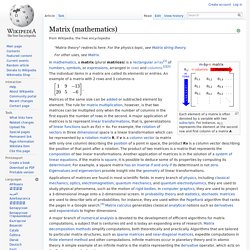
For instance, a2,1 represents the element at the second row and first column of a matrix A. Applications of matrices are found in most scientific fields. In every branch of physics, including classical mechanics, optics, electromagnetism, quantum mechanics, and quantum electrodynamics, they are used to study physical phenomena, such as the motion of rigid bodies.
In computer graphics, they are used to project a 3-dimensional image onto a 2-dimensional screen. In probability theory and statistics, stochastic matrices are used to describe sets of probabilities; for instance, they are used within the PageRank algorithm that ranks the pages in a Google search.[4] Matrix calculus generalizes classical analytical notions such as derivatives and exponentials to higher dimensions. The numbers, symbols or expressions in the matrix are called its entries or its elements. Matrices are commonly written in box brackets: