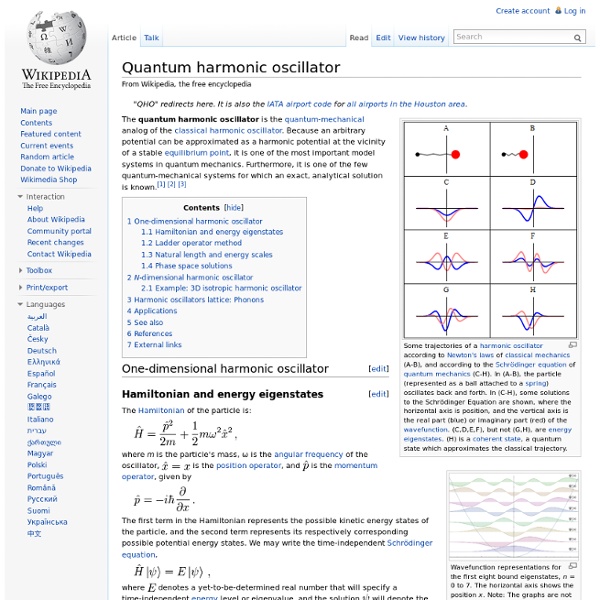
Finite potential well
The finite potential well (also known as the finite square well) is a concept from quantum mechanics. It is an extension of the infinite potential well, in which a particle is confined to a box, but one which has finite potential walls. Unlike the infinite potential well, there is a probability associated with the particle being found outside the box. Particle in a 1-dimensional box[edit] For the 1-dimensional case on the x-axis, the time-independent Schrödinger equation can be written as: where is Planck's constant, is the mass of the particle, is the (complex valued) wavefunction that we want to find, is a function describing the potential energy at each point x, and is the energy, a real number, sometimes called eigenenergy. For the case of the particle in a 1-dimensional box of length L, the potential is zero inside the box, but rises abruptly to a value at x = -L/2 and x = L/2. Inside the box[edit] For the region inside the box V(x) = 0 and Equation 1 reduces to Letting the equation becomes .
Particle in a box
In quantum mechanics, the particle in a box model (also known as the infinite potential well or the infinite square well) describes a particle free to move in a small space surrounded by impenetrable barriers. The model is mainly used as a hypothetical example to illustrate the differences between classical and quantum systems. In classical systems, for example a ball trapped inside a large box, the particle can move at any speed within the box and it is no more likely to be found at one position than another. The particle in a box model provides one of the very few problems in quantum mechanics which can be solved analytically, without approximations. One-dimensional solution[edit] The barriers outside a one-dimensional box have infinitely large potential, while the interior of the box has a constant, zero potential. The simplest form of the particle in a box model considers a one-dimensional system. where is the length of the box and is the position of the particle within the box. and . .
Macroscopic quantum phenomena
Quantum mechanics is most often used to describe matter on the scale of molecules, atoms, or elementary particles. However some phenomena, particularly at low temperatures, show quantum behavior on a macroscopic scale. The best-known examples of macroscopic quantum phenomena are superfluidity and superconductivity; another example is the quantum Hall effect. Between 1996 to 2003 four Nobel prizes were given for work related to macroscopic quantum phenomena.[1] Macroscopic quantum phenomena can be observed in superfluid helium and in superconductors,[2] but also in dilute quantum gases and in laser light. Quantum phenomena are generally classified as macroscopic when the quantum states are occupied by a large number of particles (typically Avogadro's number) or the quantum states involved are macroscopic in size (up to km size in superconducting wires). Consequences of the macroscopic occupation[edit] Fig.1 Left: only one particle; usually the small box is empty. with Ψ₀ the amplitude and
Solution of Schrödinger equation for a step potential
In quantum mechanics and scattering theory, the one-dimensional step potential is an idealized system used to model incident, reflected and transmitted matter waves. The problem consists of solving the time-independent Schrödinger equation for a particle with a step-like potential in one dimension. Typically, the potential is modelled as a Heaviside step function. Calculation[edit] Schrödinger equation and potential function[edit] Scattering at a finite potential step of height V0, shown in green. The time-independent Schrödinger equation for the wave function is The barrier is positioned at x = 0, though any position x0 may be chosen without changing the results, simply by shifting position of the step by −x0. The first term in the Hamiltonian, is the kinetic energy of the particle. Solution[edit] The step divides space in two parts: x < 0 and x > 0. both of which have the same form as the De Broglie relation (in one dimension) Boundary conditions[edit] Transmission and reflection[edit]
Free particle
Density matrix
Explicitly, suppose a quantum system may be found in state with probability p1, or it may be found in state with probability p2, or it may be found in state with probability p3, and so on. By choosing a basis (which need not be orthogonal), one may resolve the density operator into the density matrix, whose elements are[1] For an operator (which describes an observable is given by[1] In words, the expectation value of A for the mixed state is the sum of the expectation values of A for each of the pure states Mixed states arise in situations where the experimenter does not know which particular states are being manipulated. Pure and mixed states[edit] In quantum mechanics, a quantum system is represented by a state vector (or ket) . is called a pure state. and a 50% chance that the state vector is . A mixed state is different from a quantum superposition. Example: Light polarization[edit] The incandescent light bulb (1) emits completely random polarized photons (2) with mixed state density matrix .
Matrix mechanics
Matrix mechanics is a formulation of quantum mechanics created by Werner Heisenberg, Max Born, and Pascual Jordan in 1925. In some contrast to the wave formulation, it produces spectra of energy operators by purely algebraic, ladder operator, methods.[1] Relying on these methods, Pauli derived the hydrogen atom spectrum in 1926,[2] before the development of wave mechanics. Development of matrix mechanics[edit] In 1925, Werner Heisenberg, Max Born, and Pascual Jordan formulated the matrix mechanics representation of quantum mechanics. Epiphany at Helgoland[edit] In 1925 Werner Heisenberg was working in Göttingen on the problem of calculating the spectral lines of hydrogen. "It was about three o' clock at night when the final result of the calculation lay before me. The Three Fundamental Papers[edit] After Heisenberg returned to Göttingen, he showed Wolfgang Pauli his calculations, commenting at one point:[4] In the paper, Heisenberg formulated quantum theory without sharp electron orbits. W.