


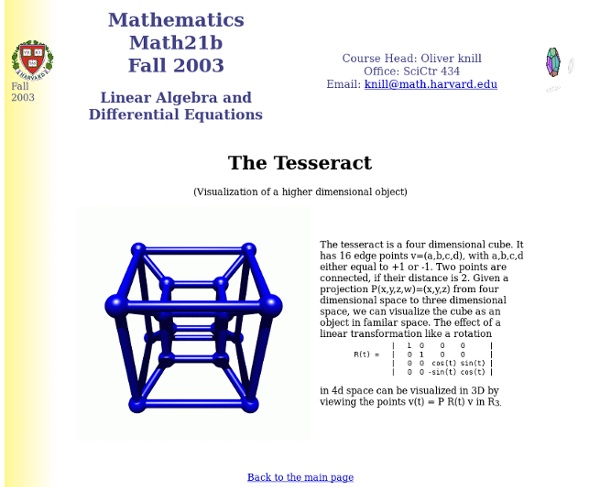
http://www.math.harvard.edu/archive/21b_fall_03/4dcube/
Nerd Paradise : Divisibility Rules for Arbitrary Divisors It's rather obvious when a number is divisible by 2 or 5, and some of you probably know how to tell if a number is divisible by 3, but it is possible to figure out the division 'rule' for any number. Here are the rules for 2 through 11... The last digit is divisible by 2. The sum of all the digits in the number is divisible by 3. The last 2 digits are divisible by 4. The last digit is 5 or 0. Physics Various examples of physical phenomena Physics is one of the oldest academic disciplines, perhaps the oldest through its inclusion of astronomy.[8] Over the last two millennia, physics was a part of natural philosophy along with chemistry, certain branches of mathematics, and biology, but during the Scientific Revolution in the 17th century, the natural sciences emerged as unique research programs in their own right.[b] Physics intersects with many interdisciplinary areas of research, such as biophysics and quantum chemistry, and the boundaries of physics are not rigidly defined. New ideas in physics often explain the fundamental mechanisms of other sciences[6] while opening new avenues of research in areas such as mathematics and philosophy.
8 math talks to blow your mind Mathematics gets down to work in these talks, breathing life and logic into everyday problems. Prepare for math puzzlers both solved and unsolvable, and even some still waiting for solutions. Ron Eglash: The fractals at the heart of African designs When Ron Eglash first saw an aerial photo of an African village, he couldn’t rest until he knew — were the fractals in the layout of the village a coincidence, or were the forces of mathematics and culture colliding in unexpected ways? Here, he tells of his travels around the continent in search of an answer. How big is infinity? There are more whole numbers than there are even numbers … right? Weierstrass functions Weierstrass functions are famous for being continuous everywhere, but differentiable "nowhere". Here is an example of one: It is not hard to show that this series converges for all x.
Mathematics Field of study Mathematics (from Greek: μάθημα, máthēma, 'knowledge, study, learning') includes the study of such topics as quantity (number theory),[1] structure (algebra),[2] space (geometry),[1] and change (analysis).[3][4][5] It has no generally accepted definition.[6][7] Rigorous arguments first appeared in Greek mathematics, most notably in Euclid's Elements.[10] Since the pioneering work of Giuseppe Peano (1858–1932), David Hilbert (1862–1943), and others on axiomatic systems in the late 19th century, it has become customary to view mathematical research as establishing truth by rigorous deduction from appropriately chosen axioms and definitions. Mathematics developed at a relatively slow pace until the Renaissance, when mathematical innovations interacting with new scientific discoveries led to a rapid increase in the rate of mathematical discovery that has continued to the present day. History
- StumbleUpon The length of the polygonal spiral is found by noting that the ratio of inradius to circumradius of a regular polygon of sides is The total length of the spiral for an -gon with side length is therefore Nerd Paradise : Calculating Base 10 Logarithms in Your Head Calculating base 10 logarithms in your head on the fly is a lot easier than you may think. It is simply a matter of memorization and a little estimation... First memorize all the single digit base 10 logs. Don't worry, it's not as painful as it sounds. I even made the chart for you: Remember this rule from high school?
New Mayan calendar discovered: world won't end in 2012 Earth has a new reason to celebrate. It's looking like we will make it past Dec. 21, 2012. According to LiveScience, researchers have unearthed the oldest-known version of the ancient Maya calendar in the Guatemalan rainforest. Archaeologist David Stuart of the University of Texas, who worked to decipher the glyphs, told LiveScience the calendar does not mark the end of the world. In fact, quite the opposite. Stuart said, "The Mayan calendar is going to keep going for billions, trillions, octillions of years into the future. from Wolfram MathWorld Poker is a card game played with a normal deck of 52 cards. Sometimes, additional cards called "jokers" are also used. In straight or draw poker, each player is normally dealt a hand of five cards. Depending on the variant, players then discard and redraw cards, trying to improve their hands.
Mathematical Atlas: A gateway to Mathematics Welcome! This is a collection of short articles designed to provide an introduction to the areas of modern mathematics and pointers to further information, as well as answers to some common (or not!) questions. The material is arranged in a hierarchy of disciplines, each with its own index page ("blue pages").
Methods for Studying Coincidences One of my favorite mathematics papers of all time is called “Methods for Studying Coincidences.” By Persi Diaconis and Frederick Mosteller, it aims to provide a rigorous mathematical framework for the study of coincidences. Using probabilistic analysis, the paper explores everything from why we see newly learned words almost immediately after first learning them, to why double lottery winners exist, to even the frequency of meeting people with the same birthday. They even explore whether or not we can statistically state that Shakespeare used alliteration, or if the frequency of words with similar-sounding beginnings could simply be explained by chance alone. For example, when it comes to newly learned words, we are often astonished that as soon as we learn a new word, we begin to see it quite frequently, or at least soon after we learn it.
An Overview of Cryptography As an aside, the AES selection process managed by NIST was very public. A similar project, the New European Schemes for Signatures, Integrity and Encryption (NESSIE), was designed as an independent project meant to augment the work of NIST by putting out an open call for new cryptographic primitives. NESSIE ran from about 2000-2003.