Diaphragm (optics)
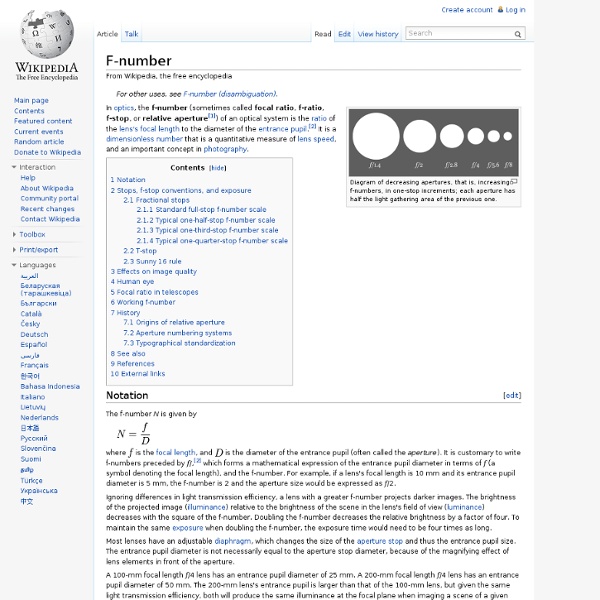
A 35 mm lens set to f/8; the diameter of the seven-sided entrance pupil, the virtual image of the opening in the iris diaphragm, is 4.375 mm Most modern cameras use a type of adjustable diaphragm known as an iris diaphragm, and often referred to simply as an iris. See the articles on aperture and f-number for the photographic effect and system of quantification of varying the opening in the diaphragm. Nine-blade iris Pentacon 2.8/135 lens with 15-blade iris In the early years of photography, a lens could be fitted with one of a set of interchangeable diaphragms [1], often as brass strips known as Waterhouse stops or Waterhouse diaphragms. The diaphragm usually has two to eight blades, depending on price and quality of the device in which it is used. In case of an even number of blades, the two spikes per blade will overlap each other, so the number of spikes visible will be the number of blades in the diaphragm used. In 1867, Dr. * In optics, stop and diaphragm are synonyms.
Het verschil tussen full-frame en crop sensor - Maarten van de Kamp
Full-frame sensoren worden gebruikt in het duurste en meest professionele segment van fotografie, waar crop sensoren, zoals de APS-C sensor, ingebouwd worden in de instap en semi-professionele segmenten van fotografie. Dit artikel gaat specifiek over de verschillen tussen de full frame sensor en een sensor met een cropfactor. Over de bijbehorende objectieven kun je lezen in dit artikel. Full-frame sensor Een full-frame sensor is een sensor met de afmetingen van 36 x 24 mm (breedte x hoogte). De reden dat dit full-frame heet is om dat dit formaat overeenkomt met de afmetingen van fotorolletjes. Voordelen t.o.v. crop sensor Een full-frame sensor heeft als voordeel dat er meer ruimte is tussen de gevoelige pixels waardoor er minder kans is op ruisvorming. Een voorbeeld van deze scherptediepte: Een Canon EOS 5D Mk II met een 70 – 200 f/2.8 lens kan over het gehele bereik een diafragma van f/2.8 gebruiken. Crop sensor Voordelen t.o.v. full-frame Het concrete verschil Het verschil met voorbeelden:
Entrance pupil
A camera lens adjusted for large and small aperture. The entrance pupil is the image of the physical aperture, as seen through the front of the lens. The size and location may differ from those of the physical aperture, due to magnification by the lens. The apparent location of the anatomical pupil of a human eye (black circle) is the eye's entrance pupil. In an optical system, the entrance pupil is the optical image of the physical aperture stop, as 'seen' through the front of the lens system. The entrance pupil of the human eye, which is not quite the same as the physical pupil, is typically about 4 mm in diameter. See also[edit] References[edit] Jump up ^ Greivenkamp, John E. (2004). External links[edit] Stops and Pupils in Field Guide to Geometrical Optics Greivenkamp, John E, 2004
Composities met kleur | DIGITALE•FOTOGRAFIETIPS
Kleur is een enorm belangrijk compositie element in je foto’s. Kleur kan een emotionele reactie van de kijker uitlokken en voegt een visuele impact toe aan de foto. Kleuren zijn diepgeworteld in de visuele cultuur. Met kleur grijp je de aandacht van de kijker, felle kleuren doen het altijd goed. Canon EOS 5DmkII, 73mm, 1/125s op f/4, ISO 1600 Maar een andere belangrijke functie van kleur in je compositie is om een sfeergevoel mee te geven door middel van het kleurgebruik en dan kan kleur juist heel subtiel worden ingezet. Door aandacht te besteden aan deze twee functies van kleur in je composities stijgt je foto boven de middenmoot uit. Eigenschappen van kleur Kleur heeft een aantal eigenschappen die van belang zijn voor het beoordelen van de toepassing. Hue geeft de kleuren van het kleurenspectrum aan. De kleurverzadiging geeft aan hoeveel kleur er aanwezig is. Licht geeft aan waar de tint of toon van een kleur zit tussen de twee uitersten van zwart en wit. Licht Kleurverzadiging Blauw Groen
Aperture
A large (1) and a small (2) aperture Aperture mechanism of Canon 50mm f/1.8 II lens, with 5 blades Definitions of Aperture in the 1707 Glossographia Anglicana Nova[1] In some contexts, especially in photography and astronomy, aperture refers to the diameter of the aperture stop rather than the physical stop or the opening itself. For example, in a telescope the aperture stop is typically the edges of the objective lens or mirror (or of the mount that holds it). Sometimes stops and diaphragms are called apertures, even when they are not the aperture stop of the system. Application[edit] The aperture stop is an important element in most optical designs. The size of the stop is one factor that affects depth of field. In addition to an aperture stop, a photographic lens may have one or more field stops, which limit the system's field of view. The biological pupil of the eye is its aperture in optics nomenclature; the iris is the diaphragm that serves as the aperture stop. In photography[edit]
Neutral density filter
Demonstration of the effect of a neutral density filter In photography and optics, a neutral density filter or ND filter is a filter that reduces or modifies the intensity of all wavelengths or colors of light equally, giving no changes in hue of color rendition. It can be a colorless (clear) or grey filter. For example, one might wish to photograph a waterfall at a slow shutter speed to create a deliberate motion blur effect. Mechanism[edit] For an ND filter with optical density d the amount of optical power transmitted through the filter, which can be calculated from the logarithm of the ratio of the measurable intensity (I) after the filter to the incident intensity (I0),[1] shown as the following: Fractional Transmittance (I⁄I0) = 10-d, or Uses[edit] Comparison of two pictures showing the result of using a ND-filter at a landscape. Neutral density filters are often used to achieve motion blur effects with slow shutter speeds Examples of this use include: Varieties[edit] See also[edit]