


Making a dream date - Dream Gates "At the Foothills of Mt Helen". B.K.Connelly, 1981 You’re separated from your sweetheart and you’d like to have some good private time together. Can you do that? If you are embarking on shared dreaming as home entertainment, you get to choose your category. I know what I am talking about. Want to try this? But shared dreaming doesn’t require you to start out from the same place, or even on the same continent. To keep this simple, let’s assume you have a friend who is not physically present, with whom you’d like to share a dream adventure. 1. You might simply agree to try to meet in your dreams on (say) Wednesday night. 2. If you’re new to this kind of thing, it’s probably best to start out with a place in the physical world that one or both of you know. 3. The idea of simply hanging out with your partner in a delightful locale – and not having to pay for the plane ticket or the five-star hotel suite – may be juicy enough. 4. 5. 6. When you share, be alert to the time-slip phenomenon.
Curse of dimensionality The curse of dimensionality refers to various phenomena that arise when analyzing and organizing data in high-dimensional spaces (often with hundreds or thousands of dimensions) that do not occur in low-dimensional settings such as the three-dimensional physical space of everyday experience. The term curse of dimensionality was coined by Richard E. Bellman when considering problems in dynamic optimization.[1][2] The "curse of dimensionality" depends on the algorithm[edit] The "curse of dimensionality" is not a problem of high-dimensional data, but a joint problem of the data and the algorithm being applied. When facing the curse of dimensionality, a good solution can often be found by changing the algorithm, or by pre-processing the data into a lower-dimensional form. Curse of dimensionality in different domains[edit] Combinatorics[edit] , exponential in the dimensionality. Sampling[edit] Optimization[edit] Machine learning[edit] Bayesian statistics[edit] Distance functions[edit] and dimension .
Marqueed. Discuter autour d'une image avec la classe. Marqueed est un outil en ligne qui permet d’échanger, de travailler et de discuter autour d’une image à plusieurs. Le concept de marqueed est assez simple à comprendre. Il permet sur une sorte de tableau blanc virtuel de discuter à plusieurs autour d’une image. Une fois votre image installée sur l’espace de travail en ligne de Marqueed, vous allez pouvoir y insérer des points de discussion dans lesquels vous pourrez apporter vos commentaires sous forme de texte. Il ne vous restera plus qu’a inviter un ou plusieurs étudiants à collaborer sur une ou plusieurs images, il suffira de les inviter par email. Dans la classe. Marqueed est un outil de travail collaboratif qui peut être utile pour proposer un document à lire à une classe avec vos commentaires et annotations. Marqueed fonctionne avec la pluspart des formats d’images mais aussi avec des fichiers pdf ce qui permettra d’être utilisé avec la plupart des fichiers utiles pour la classe. Le service est gratuit. Sur le même thème
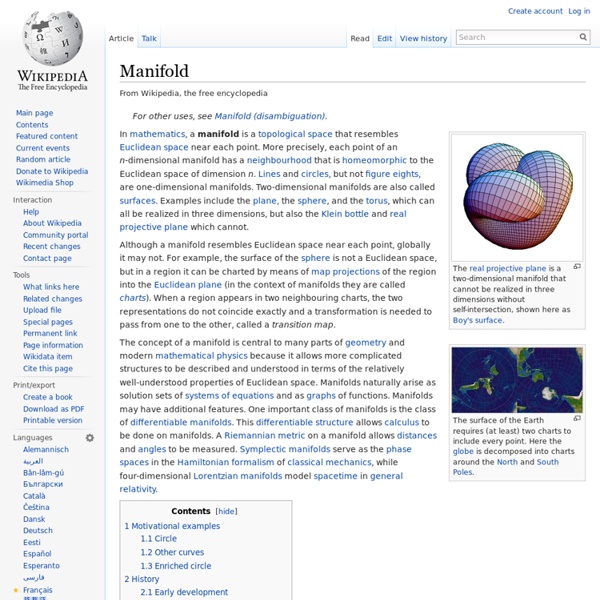
Vector space Vector addition and scalar multiplication: a vector v (blue) is added to another vector w (red, upper illustration). Below, w is stretched by a factor of 2, yielding the sum v + 2w. An example of a vector space is that of Euclidean vectors, which may be used to represent physical quantities such as forces: any two forces (of the same type) can be added to yield a third, and the multiplication of a force vector by a real multiplier is another force vector. In the same vein, but in a more geometric sense, vectors representing displacements in the plane or in three-dimensional space also form vector spaces. Vectors in vector spaces do not necessarily have to be arrow-like objects as they appear in the mentioned examples: vectors are best thought of as abstract mathematical objects with particular properties, which in some cases can be visualized as arrows. Introduction and definition[edit] The concept of vector space will first be explained by describing two particular examples: and
Master Villes, Services, Usages (VISU) Contexte La vie en ville repose aujourd’hui sur une pluralité de services. Historiquement ces services ont été : la fourniture d’eau, d’électricité ou de gaz, l’assainissement, la téléphonie, l’accès à un réseau de transport, la poste. Plus récemment l’accès au câble ou à Internet est venu s’ajouter à cette liste. On parle également, de plus en plus, de services à domicile. Les municipalités élargissent leur gamme d’intervention auprès de leurs administrés. Tous ces services sont fournis par des gestionnaires publics ou privés qui font face à des usagers de plus en plus exigeants et divers. C’est dans ce contexte que cette spécialité entend former des spécialistes qui seront à même d’articuler analyse des usages et conception de services au sein d’une pratique d’aménagement. Cette spécialité est co-habilitée par l'Université Paris-Est Marne-la-Vallée et l'École des Ponts ParisTech. Objectifs Débouchés Ils concernent principalement les métiers de l’étude et du conseil :
Dimensions in Philosophy Mathematics and Reality: Is Mathematics a symbolic Universe Invented by the Human Mind? (part 1 of 5) | Poetic Mind by Paul Hartal. Introduction Mathematics is a model of exact reasoning, the most precise branch of human knowledge. Drawing on the mathematical genius of such giants as Euler, Lobachevski, Riemann, Russell and Einstein; I shall explore in this article an array of inherent contradictions in the logical foundations of the Queen of Sciences and discuss some major mathematical earthquakes that shook its theoretical bedrock. Chapter 1: An infallible delegate of truth? Mathematics undoubtedly represents a crowning accomplishment of the human intellect but does it correspond to the material world? In contrast to this, the philosopher Bertrand Russell in 1901 uttered that “Mathematics may be defined as the subject matter in which we never know what we are talking about, nor whether what we are saying is true” (3). Figure 1: ‘The Mathematician’, Acrylic on canvas, 60 cm x 45 cm, 2003 (Collection of Hanseo University Art Museum, Seoul). Figure 2: ‘Oh La la’, Acrylic on canvas, 60 cm x 45 cm, 2009.
Fourth dimension in literature The idea of a fourth dimension has been a factor in the evolution of modern art, but use of concepts relating to higher dimensions has been little discussed by academics in the literary world.[1] From the late 1800s onwards, many writers began to make use of possibilities opened up by the exploration of such concepts as hypercubes and non-Euclidian geometry. While many writers took the fourth dimension to be one of time (as it is commonly considered today), others preferred to think of it in spatial terms, and some associated the new mathematics with wider changes in modern culture. Early influence[edit] Theoretical physicist James Clerk Maxwell is best known for his work in formulating the equations of electromagnetism. He was also a prize-winning poet,[2] and in his last poem Paradoxical Ode; Maxwell muses on connections between science, religion and nature, touching upon higher-dimensions along the way:[3] "..shall we stay our upward course? H.G. Other works[edit] References[edit]
Meograph. Creer des histoires multimedia Meograph est un outil tice en ligne qui permet de créer des histoires multimédia facilement. Meograph est un outil de « storytelling » qui met à la portée d’un enseignant ou d’un élève la création d’un clip qui associe du son, une frise chronologique, une carte, et des illustrations qui peuvent être des photos ou des vidéos. C’est pour cela que Méograph se présente comme créateur d’histoires en quatre dimensions. Le principal atout de cet outil gratuit, c’est sa simplicité d’utilisation. On crée une histoire en suivant un pas-à-pas proposé par la plateforme. Méograph vous propose un enregistrement directement depuis son interface, mais vous pouvez aussi uploader un Mp3. Dans la classe. Meograph est un outil formidable pour créer facilement une leçon ou une présentation multimédia susceptible de retenir l’attention des élèves grâce au mix numérique d’images et de vidéos. Meograph pour l’éducation est gratuit et mérite amplement que vous le testiez. Lien : Meograph. Sur le même thème
Networks and dimension Mesure (mathématiques) Un article de Wikipédia, l'encyclopédie libre. Pour les articles homonymes, voir Mesure. De façon informelle, une mesure a la propriété d'être monotone : si l'ensemble E est un sous-ensemble de F, la mesure de E est inférieure ou égale à celle de F. De plus, on impose à la mesure de l'ensemble vide la valeur 0. L'étude des espaces munis de mesures est l'objet de la théorie de la mesure. de parties de X une valeur μ(S), qui est un réel positif ou l'infini. Définition — Soit un espace mesurable (i.e. un couple où est un ensemble et Une application μ définie sur à valeurs dans est appelée mesure lorsque les deux propriétés suivantes sont satisfaites : on parle de mesure -finie[4]. par on peut supposer que la suite de sous-ensembles figurant dans la définition est croissante pour l'inclusion[5]. Un sous-ensemble S de X est dit négligeable lorsqu'il est inclus dans un T appartenant à la tribu et de mesure nulle[6].La mesure μ est dite complète lorsque tout ensemble négligeable appartient à la tribu [7].