Transformation of Trigonometric Graphs
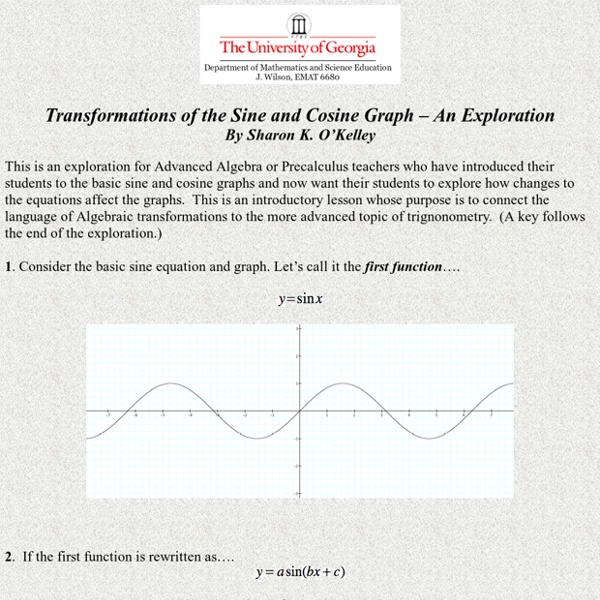
OML Search In these lessons, we will learn how Trigonometric Graphs can be transformed. the amplitude and vertical shift of Trigonometric Graphs the period and phase shift of Trigonometric Graphs Related Topics:More Trigonometric Lessons Stretching and Compressing of Graphs Amplitude of Trigonometric Functions
Graphs of trigonometric functions
The Topics | Home Zeros of a function The graph of y = sin x The period of a function
Questions on Trigonometric Functions
1. Use the definitions of the six trigonometric functions and the Pythagorean Identity given in the Field Guide Lesson to show that: 1 + tan2(x) = sec2(x) 1 + cot2(x) = csc2(x)
Radians to degrees
How to convert degrees to radians or radians to degrees. Theory: What are 'radians' ? One radian is the angle of an arc created by wrapping the radius of a circle around its circumference. In this diagram, the radius has been wrapped around the circumference to create an angle of 1 radian.
Review : Trig Functions
The intent of this section is to remind you of some of the more important (from a Calculus standpoint…) topics from a trig class. One of the most important (but not the first) of these topics will be how to use the unit circle. We will actually leave the most important topic to the next section. First let’s start with the six trig functions and how they relate to each other.
How to Change the Amplitude, Period, and Position of a Tangent or Cotangent Graph
You can transform the graph for tangent and cotangent vertically, change the period, shift the graph horizontally, or shift it vertically. However, you should take each transformation one step at a time. For example, to graph
Basic Trigonometric Graphs
Trigonometric Functions and Their Graphs: The Sine and Cosine (page 1 of 3) Sections: The sine and cosine, The tangent, The co-functions At first, trig ratios related only to right triangles. Then you learned how to find ratios for any angle, using all four quadrants. Then you learned about the unit circle, in which the value of the hypotenuse was always r = 1 so that sin(θ) = y and cos(θ) = x.
Scaffolded Math and Science: Middle School Math Word Wall Ideas
For a year between teaching mainstream high school math and teaching special education high school math, I tried teaching middle school math. My husband and I had moved further out from the city, the job was listed as an 8th grade Algebra position, I love Algebra, it was closer to my new apartment, what could go wrong?Everything.
TESOL Event Detail - TESOL Core Certificate Program (Cohort 22) - More About the Program
Set yourself apart and teach almost anywhere in the world with a certificate from TESOL International Association, the globally-recognized trusted provider of ELT professional learning. The TESOL Core Certificate Program (TCCP) is aligned to TESOL’s Standards for Short-Term TEFL/TESL Certificate Programs and was developed by experts in the TESOL field. Gain foundational knowledge in the theory and practice of English language teaching (ELT), focus your skills on teaching adult or young learners, and apply what you’ve learned through an in-person or virtual teaching practicum.
Understanding Language
This eighth grade (middle school) task provides students opportunities to interpret a situation, represent the variables mathematically, select appropriate mathematical methods, interpret and evaluate the data generated, and communicate their reasoning. Students work with selected formulas to model a situation, interpret given data, make approximations, communicate their reasoning in verbal and written form, and critique solutions developed by others. This task draws on understandings of rate and proportional reasoning (a CCSS focus of grades 6 and 7), geometric measurement and volume (which begins with right rectangular prisms in grade 5, and is extended in grades 6, 7, and 8), and builds toward the high school number and quantity standard of interpreting units consistently in formulas. Making Matchsticks is part of a Formative Assessment Lesson (FAL), which can be downloaded here:
NumberFix: RAFT Writing Prompts for Math
Writing Across the Curriculum: R.A.F.T. Prompts for Math Class building a writing prompt that challenges students to think deeply about math Classroom writing assignments can feel very unauthentic to our students.
A Guide to the 8 Mathematical Practice Standards
Common Core mathematics is a way to approach teaching so that students develop a mathematical mindset and see math in the world around them. We are making problem-solvers. No matter what your objectives, textbook, or grade level, the eight mathematical practice standards are a guide to good math instruction. Here they are in plain English with suggestions for incorporating them into your everyday math class.
common core resources /mathematical practice standards /standard 2: reason abstractly & quantitatively /
classroom observations: Teachers who are developing students’ capacity to "reason abstractly and quantitatively" help their learners understand the relationships between problem scenarios and mathematical representation, as well as how the symbols represent strategies for solution. A middle childhood teacher might ask her students to reflect on what each number in a fraction represents as parts of a whole. A different middle childhood teacher might ask his students to discuss different sample operational strategies for a patterning problem, evaluating which is the most efficient and accurate means of finding a solution. Visit the video excerpts below to view these teachers engaging their students in abstract and quantitative reasoning. the standard: Mathematically proficient students make sense of quantities and their relationships in problem situations.