

Journal of Theoretical Biology : The promise of Mechanical Turk: How online labor markets can help theorists run behavioral experiments. Volume 299, 21 April 2012, Pages 172–179 Evolution of Cooperation Edited By Martin Nowak Abstract Combining evolutionary models with behavioral experiments can generate powerful insights into the evolution of human behavior.

The emergence of online labor markets such as Amazon Mechanical Turk (AMT) allows theorists to conduct behavioral experiments very quickly and cheaply. Keywords Evolutionary game theory; Experimental economics; Cooperation; Internet; Economic games Copyright © 2011 Elsevier Ltd. Iowa Electronic Markets. Iowa Electronic Market for 2008 Democratic National Primary.

The Obama spike in February is a result of Super Tuesday. The Iowa Electronic Markets (IEM) are a group of real-money prediction markets/futures markets operated by the University of Iowa Tippie College of Business. Unlike normal futures markets, the IEM is not-for-profit; the markets are run for educational and research purposes. The IEM allows traders to buy and sell contracts based on, among other things, political election results and economic indicators.
Some markets are only available to academic traders. The IEM has often been used to predict the results of political elections with a greater accuracy than traditional polls.[1][2][3][4] A precursor to the IEM was the Iowa Political Stock Market (IPSM), invented by George Neumann, and was developed by Robert E. How it works[edit] Here are examples of contracts that the IEM traded, beginning June 6, 2006, concerning the 2008 U.S. Rules and limits[edit] See also[edit] Prediction market. People who buy low and sell high are rewarded for improving the market prediction, while those who buy high and sell low are punished for degrading the market prediction.
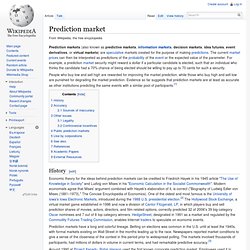
Evidence so far suggests that prediction markets are at least as accurate as other institutions predicting the same events with a similar pool of participants.[1] History[edit] Prediction markets have a long and colorful lineage. Betting on elections was common in the U.S. until at least the 1940s, with formal markets existing on Wall Street in the months leading up to the race. Newspapers reported market conditions to give a sense of the closeness of the contest in this period prior to widespread polling. Around 1990 at Project Xanadu, Robin Hanson used the first known corporate prediction market. In July 2003, the U.S. Knapsack problem. Example of a one-dimensional (constraint) knapsack problem: which boxes should be chosen to maximize the amount of money while still keeping the overall weight under or equal to 15 kg?
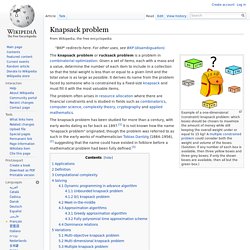
A multiple constrained problem could consider both the weight and volume of the boxes. (Answer: if any number of each box is available, then three yellow boxes and three grey boxes; if only the shown boxes are available, then all but the green box.) The knapsack problem or rucksack problem is a problem in combinatorial optimization: Given a set of items, each with a mass and a value, determine the number of each item to include in a collection so that the total weight is less than or equal to a given limit and the total value is as large as possible.
It derives its name from the problem faced by someone who is constrained by a fixed-size knapsack and must fill it with the most valuable items. Applications[edit] Definition[edit] Mathematically the 0-1-knapsack problem can be formulated as: Let there be items, to.
Optimization problem. Continuous optimization problem[edit] The standard form of a (continuous) optimization problem is[1] where is the objective function to be minimized over the variable , are called inequality constraints, and are called equality constraints.
By convention, the standard form defines a minimization problem. A maximization problem can be treated by negating the objective function. Combinatorial optimization problem[edit] Formally, a combinatorial optimization problem is a quadruple , where is a set of instances;given an instance , is the set of feasible solutions;given an instance and a feasible solution of , denotes the measure of , which is usually a positive real. is the goal function, and is either or . The goal is then to find for some instance an optimal solution, that is, a feasible solution with For each combinatorial optimization problem, there is a corresponding decision problem that asks whether there is a feasible solution for some particular measure which contains vertices and to.