

64 tetrahedron grid. Star tetrahedron infinite division. Birth Into 6th Dimension (Sacred Geometry by ieoie) Nassim Haramein - Sacred Geometry and Unified Fields. Nassim Haramein - Tree of Life. Nassim Haramein Cognos 2010 - ENGLISH PART 1 OF 6. Vector Equilibrium & Isotropic Vector Matrix. As has been stated throughout this website, the Vector Equilibrium (VE) is the most primary geometric energy array in the cosmos.
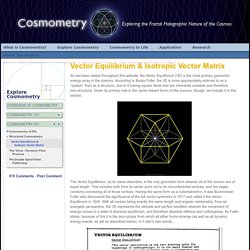
According to Bucky Fuller, the VE is more appropriately referred to as a “system” than as a structure, due to it having square faces that are inherently unstable and therefore non-structural. Given its primary role in the vector-based forms of the cosmos, though, we include it in this section. The Importance of 432Hz Music. Article By: Brian T Collins Can the current international concert pitch of music somehow be improved to create a more resonant and pleasant positive experience for both the musician and the listener?
Can that change be more resonant based on observations of geometry and mathematical patterns found in nature? To answer these important questions we first must look at natural design and how we can apply that to music. Our inner ear for example works on the basis of Phi dampening.
VideoLightBox Gallery generated by VideoLightBox.com. Sacred geometry. Pyramids of Gizeh as an alternative energy source on Earth. As I go through the "mental organizing" of this site, I realize more and more that I must format this information immediately before it gets out of hand.

There is an unlimited amount of information in the hieroglyphics explaining the quantum physics that was a part of the "The Ancients" average daily life. Because of the keen interest this site is generating in the short time it has existed, I have decided to make the effort to place new information onto it weekly. I will show you a new way to interpret the language of geometry on the hieroglyphics. The geometry in hieroglyphics reflects the geometry in nature and this geometry explains quantum physics. Quantum physics explains how we are all connected to one another beginning with the most minute of all particles; a photon particle. From this day on additional information will be placed at the "END" of this site. PS. A FEW MORE SYMBOLS TO THINK ABOUT (December 18, 2007) We observe Mother Nature altering gravity every day.
Keep it Simple. Magic square. It is possible to construct a normal magic square of any size except 2 × 2 (that is, where n = 2), although the solution to a magic square where n = 1 is trivial, since it consists simply of a single cell containing the number 1.
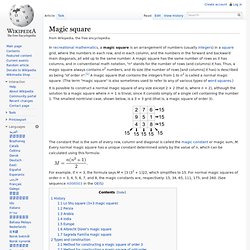
The smallest nontrivial case, shown below, is a 3 × 3 grid (that is, a magic square of order 3). The constant that is the sum of every row, column and diagonal is called the magic constant or magic sum, M. Every normal magic square has a unique constant determined solely by the value of n, which can be calculated using this formula: For example, if n = 3, the formula says M = [3 (32 + 1)]/2, which simplifies to 15.
For normal magic squares of order n = 3, 4, 5, 6, 7, and 8, the magic constants are, respectively: 15, 34, 65, 111, 175, and 260. History[edit]
Welcome to Math Craft World! (Bonus: How to Make Your Own Paper Polyhedra) 7 Templates for Slide-Together Geometric Paper Constructions. The "slide-together" paper construction method is a fun and satisfying way to build 3D geometric objects.
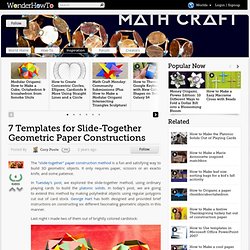
It only requires paper, scissors or an exacto knife, and some patience. In Tuesday's post, we explored the slide-together method, using ordinary playing cards to build the platonic solids. In today's post, we are going to extend this method by making polyhedral objects using regular polygons cut out of card stock. George Hart has both designed and provided brief instructions on constructing six different fascinating geometric objects in this manner.
Last night I made two of them out of brightly colored cardstock: To make these paper sculptures, you can use the template links I've provided below in pdf format. 12 Decagons Template 20 Triangles Template 12 Decagrams Template 20 Hexagons Template. Math Craft Inspiration of the Week: The Polyhedral Metal Sculptures of Vladimir Bulatov. Vladimir Bulatov makes sculptures of fantastic variations on polyhedra and other geometric objects.
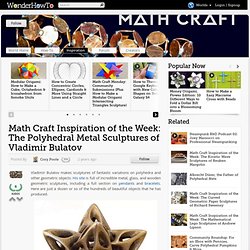
His site is full of incredible metal, glass, and wooden geometric sculptures, including a full section on pendants and bracelets. Here are just a dozen or so of the hundreds of beautiful objects that he has produced. What do you think of Vladimir's sculptures and jewelry? Does anything in this post inspire you? Comment below. Have a great weekend and if you create any math-related art, please share with all of us on the Math Craft corkboard.
Math Craft Monday: Community Submissions (Plus How to Make an Orderly Tangle of Triangles) Math Craft Monday: Community Submissions (Plus How to Make an Orderly Tangle of Triangles) It's Monday, which means once again, it's time to highlight some of the recent community submissions posted to the Math Craft corkboard.
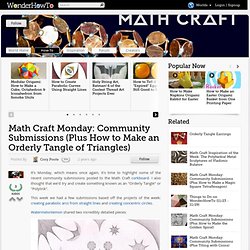
I also thought that we'd try and create something known as an "Orderly Tangle" or "Polylink". This week we had a few submissions based off the projects of the week: creating parabolic arcs from straight lines and creating concentric circles. Watermelonlemon shared two incredibly detailed pieces: Cerek Tunca extended the idea of drawing parabolic curves using straight lines by also connecting all of the lines that wouldn't cover over the curve. Justin Meyers of Scrabble world posted several pictures of a translucent cube with curve stitching designs that was colored like a rubiks cube. We also had two submissions from Imatfaal Avidya. The second submission is his recreation of Thomas Hull's Five Intersecting Tetrahedra. Materials and Tools Download the template.
Numbers.